BEFORE: Steady-State error control system
NEXT: PID – Effect of integrative and derivative control actions.
Introduction
An automatic controller compares the real value of the output of a plant with the input reference (the desired value), determines the deviation and produces a control signal that will reduce the deviation to zero or a small value. The way in which the automatic controller produces the control signal is called control action.
Classification of industrial controls
According to their control actions, industrial controllers are classified as:
- Two-position (On / Off)
- Proportional
- Integrals
- Proportional-Integrals
- Proportional-Derivatives
- Proportional-Integrals-Derivatives
Almost all industrial controllers use electricity as an energy source or a pressurized fluid, such as oil or air. The controllers can also be classified, according to the type of energy they use in their operation, like pneumatic, hydraulic or electronic. The type of controller that is used must be decided based on the nature of the plant and operational conditions, including considerations such as safety, cost, availability, reliability, precision, weight and size.
Figure 5-1 shows a typical configuration for an Industrial Control System:


The previous figure consists of a Block Diagram for an industrial control system composed of an automatic controller, an actuator, a plant and a sensor (measuring element). The controller detects the error signal, which is usually at a very low power level, and amplifies it to a sufficiently high level. The output of an automatic controller feeds an actuator that can be a pneumatic valve or an electric motor. The actuator is a power device that produces the input for The plant according to the control signal, so that the output signal approaches the reference input signal. The sensor, or measurement element, is a device that converts an output variable, such as a displacement, into another manageable variable, such as a voltage, that can be used to compare the output with the reference input signal. This element is in the feedback path of the closed-loop system. The setpoint of the controller must be converted into a reference input with the same units as the feedback signal from the sensor or from the measuring element.
Two positions control (On / Off).
In a two position control system, the acting element only has two fixed positions that, in many cases, are simply turned on and off. The On/Off control is relatively simple and cheap, which is why it is extensively used in industrial and domestic control systems.
Suppose that the output signal of the controller is u(t) and that the error signal is e(t). In the control of two positions, the signal u(t) remains at a value either maximum or minimum, depending on whether the error signal is positive or negative. In this way,

where U1 y U2 are constants. Very often, the minimum value of U2 is zero or –U1.
It is common for two-position controllers to be electrical devices, in which case an electrical valve operated by solenoids is widely used. Pneumatic proportional controllers with very high gains function as two-position controllers and are sometimes referred to as two-position pneumatic controllers.
Figures 5-3 (a) and (b) show the block diagrams for two controllers of two positions The range in which the error signal must move before the commutation is called differential gap. In Figure 5-3 (b) a differential gap is indicated. Such a gap causes the output of the controller u (t) to retain its present value until the error signal has moved slightly beyond zero. In some cases, the differential gap is the result of unintentional friction and a lost movement; however, it is often intentionally caused to avoid too frequent operation of the on and off mechanism.


Proportional control action.
For a controller with proportional control action, the relationship between the controller output u (t) and the error signal e (t) is:
![]()
or, in quantities transformed by the Laplace method:

where Kp is considered proportional gain.
Whatever the actual mechanism and the form of the operating power, the controller
proportional is, in essence, an amplifier with an adjustable gain. A block diagram of such a controller is presented in Figure 5-6.


Integral control action.
In a controller with integral control action, the value of the controller output u (t) is changed to a ratio proportional to the error signal e (t). That is to say,

O well:

where Ki is an adjustable constant. The transfer function of the integral controller is:

If the value of e (t) is doubled, the value of u (t) varies twice as fast. For an error of zero, the value of u (t) remains stationary. Sometimes, the integral control action is called adjustment control (reset). Figure 5-7 shows a block diagram of such controller.


Integral-proportional control action.
The control action of a proportional-integral controller (PI) is defined by:

or the transfer function of the controller, which is:

where Kp is the proportional gain and Ti is called integral time. Both Kp and Ti are adjustable. Integral time adjusts the integral control action, while a change in the value of Kp affects the integral and proportional parts of the control action.
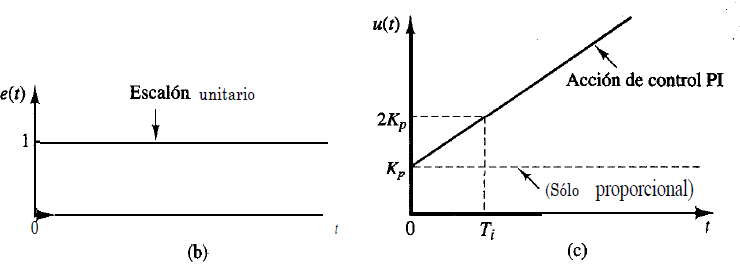
The inverse of the integral time Ti is called the readjustment speed. The rate of readjustment is the number of times per minute that the proportional part of the control action is doubled. The rate of readjustment is measured in terms of the repetitions per minute. The Figure 5-8 (a) shows a block diagram of a PI controller. If the error signal e (t) is a unit step function, as shown in Figure 5-8 (b), the controller output u (t) becomes what is shown in Figure 5-8 (c).


Proportional-derivative control action.
The control action of a proportional-derivative (PD) controller is defined by:

The transfer function is:

where Kp is the proportional gain and Td is a constant called derivative time. Both Kp and Td are adjustable. The derivative control action, sometimes called speed control, occurs where the magnitude of the controller output is proportional to the rate of change of the error signal. The derivative time Td is the time interval during which the action of the velocity advances the effect of the proportional control action.
Figure 5-9 (a) shows a block diagram of a PD controller. If the error signal e (t) is a unit ramp function as shown in Figure 5-9 (b), the controller output u (t) becomes that shown in Figure 5-9 (c). ). The derivative control action has a forecast nature. However, it is obvious that a derivative control action never foresees an action that has never occurred.


Although the derivative control action has the advantage of being forecast, it has the disadvantages that it amplifies the noise signals and can cause a saturation effect in the actuator. Note that the derivative control action is never used alone, because it is only effective during transient periods.
Proportional-Integral-derivative (PID) control action.
The combination of a proportional control action, an integral control action and a derivative control action is called proportional-integral-derivative (PID) control action.
This combined action has the advantages of each of the three individual control actions. The equation of a controller with this combined action is obtained by:

The transfer function is:

where Kp is the proportional gain, Ti is the integral time and Td is the derivative time. The block diagram of a PID controller appears in Figure 5-10 (a). If e (t) is a unit ramp function, like the one shown in Fig. 5-10 (b), the controller output u (t) becomes that of Fig. 5-10 (c).



Effects of the sensor on the performance of the system.
Since the dynamic and static characteristics of the sensor or measuring element affects the indication of the actual value of the output variable, the sensor fulfills a function important to determine the overall performance of the control system. As usual, the sensor determines the transfer function in the feedback path. If the time constants of a sensor are negligible compared to other constants of time of the control system, the sensor transfer function simply it becomes a constant. Figures 5-11 (a), (b) and (c) show diagrams of automatic controller blocks with a first-order sensor, an overdamped second-order sensor and a second-order underdamped sensor, respectively. Often the response of a thermal sensor is of the overdamped second order type.


BEFORE: Steady-State error control system
NEXT: PID – Effect of integrative and derivative control actions.
Source:
Literature review by Larry Francis Obando – Technical Specialist – Educational Content Writer
Copywriting, Content Marketing, Tesis, Monografías, Paper Académicos, White Papers (Español – Inglés)
Escuela de Ingeniería Eléctrica de la Universidad Central de Venezuela, CCs.
Escuela de Ingeniería Electrónica de la Universidad Simón Bolívar, Valle de Sartenejas.
Escuela de Turismo de la Universidad Simón Bolívar, Núcleo Litoral.
Contact: Caracas, Quito, Guayaquil, Cuenca. telf – 0998524011
WhatsApp: +593984950376
email: dademuchconnection@gmail.com

3 comentarios en “PID – Basic Control System Actions”